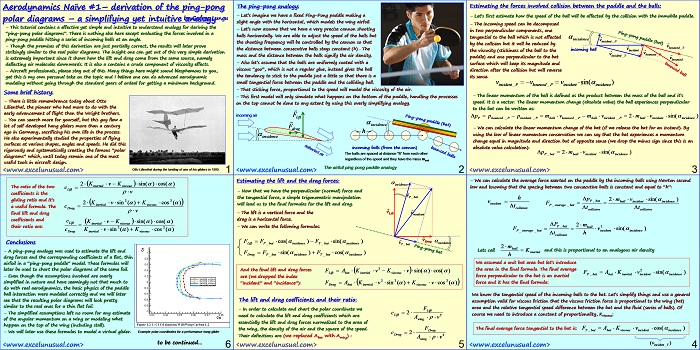
This is the ping-pong aerodynamic analogy. The wing is a ping pong bat and the air is a bunch of evenly spaced array of ping pong balls. It is a naive model but, as we will see in a later post, the polar diagrams derived from this analogy (between -12 to +12 degrees of angle of attack) are surprisingly close shape wise to the real diagrams of a thin, symmetric airfoil.
The model of course cannot possibly calculate anything related to what happens on the upper part of the foil. Later on, we will use the formulas derived here to create a 2D dynamic model of a glider and experient with things such as stability and pitch oscillation during flight, function of various position of the center of gravity and interaction with the momentum of inertia of the plane.
This tutorial is fairly easy to understand (with the proper concentration) at the high school level and can be a good casual first step in understanding general flight dynamics.
![]()
Aerodynamics Naïve #1- derivation of the ping-pong polar diagrams – a simplifying yet intuitive analogy
by George Lungu
– This tutorial contains a effective yet simple and intuitive to understand analogy for deriving the
“ping-pong polar diagrams”. There is nothing else here except evaluating the forces involved in a
ping-pong paddle hitting a series of incoming balls at an angle.
– Though the premises of this derivation are just partially correct, the results will later prove
strikingly similar to the real polar diagrams. The insight one can get out of this very simple derivation
is extremely important since it shows how the lift and drag come from the same source, namely
deflecting air molecules downwards. It is also a contains a crude component of viscosity effects.
– Aircraft professionals, please stay out of this. Many things here might sound blasphemous to you,
yet this is my own personal take on the topic and I believe one can do advanced aerodynamic
modeling without going through the standard years of ordeal for getting a minimum background.
Some brief history:
– There is little remembrance today about Otto
Lilienthal, the pioneer who had more to do with the
early advancement of flight than the Wright brothers.
– You can search more for yourself, but this guy flew a
lot of self developed hang gliders more than a century
ago in Germany, sacrificing his own life in the process.
He also experimentally studied the properties of flying
surfaces at various shapes, angles and speeds. He did this
rigorously and systematically creating the famous “polar
diagrams” which, until today remain one of the most
useful tools in aircraft design.
Otto Lilienthal during the landing of one of his gliders in 1895.
The ping-pong analogy:
– Let’s imagine we have a fixed Ping-Pong paddle making a
slight angle with the horizontal, which models the wing airfoil.
– Let’s now assume that we have a very precise cannon shooting
balls horizontally. We are able to adjust the speed of the balls but
the shooting frequency will be controlled by the cannon so that
the distance between consecutive balls stays constant (h) . The
mass and the distance between the balls signify the air density.
– Also let’s assume that the balls are uniformly coated with a
viscous “goo”, which is not a regular glue, instead gives the ball
the tendency to stick to the paddle just a little so that there is a
small tangential force between the paddle and the colliding ball.
– That sticking force, proportional to the speed will model the viscosity of the air.
– This first model will only simulate what happens on the bottom of the paddle, handling the processes
on the top cannot be done to any extent by using this overly simplifying analogy.
The balls are spaced at distance “h” from each other
regardless of the speed and they have the mass mball
The airfoil ping pong paddle analogy
Estimating the forces involved in the collision between the paddle and the balls:
– Let’s first estimate how the speed of the ball will be affected by the collision with the immobile paddle.
– The incoming speed can be decomposed
in two perpendicular components, one tangential to the bat which is not affected by the collision but it will be reduced by
incoming ball
the viscosity (stickiness of the ball to the incident
v bounced_ P
incident_T
paddle) and one perpendicular to the bat
v
bounced
surface which will keep its magnitude and
direction after the collision but will reverse
its sense.
incident_P bounced_ P incident incidence
– The linear momentum of the ball is defined as the product between the mass of the ball and it’s
speed. It is a vector. The linear momentum change (absolute value) the ball experiences perpendicular
to the bat can be written as:
P bounced_ P incident_ P ball bounced P ball incident_ P ball incident incidence
– We can calculate the linear momentum change of the bat (if we release the bat for an instant). By
using the law of linear momentum conservation we can say that the bat experiences a momentum
change equal in magnitude and direction but of opposite sense to that of the ball (we drop the minus
sign since this is an absolute value calculation): P_bat ball incident incidence
– We can calculate the average force exerted on the paddle by the incoming balls using Newton second
law and knowing that the spacing between two consecutive balls is constant and equal to “h”:
P bat ball incident incidence
incident F
P _ a vera g e_ bat
collision
P _ bat ball 2
P _ a vera g e_ bat incident incidence
Lets call K and this is proportional to an analogous air density
inertial
We assumed a unit bat area but let’s introduce
the area in the final formula. The final average 2
P_bat bat inertial incident incidence
force perpendicular to the bat is an inertial
force and it has the final formula:
We know already the tangential speed of the incoming balls to the bat. Let’s simplify things and use a
general assumption valid for viscous friction that the viscous friction force is proportional to product
of the wing (bat) area and the relative tangential speed difference between the bat and the fluid
(series of balls). Of course we need to introduce a constant of proportionality, Kviscous:
The final average force tangential to the bat is: T_bat bat viscous incidence
Estimating the lift and the drag forces:
– Now that we have the perpendicular (normal) force and the tangential force, a simple trigonometric manipulation will lead us to the final formulas for the lift and drag.
– The lift is a vertical force and the resultant drag is a horizontal force.
– We can write the following formulas:
vDrag
Lift P _ bat incidence T _ bat incidence v incidence
T bat
Drag P _ bat incidence T _ bat incidence
And the final lift and drag forces
Lift bat inertial viscous
are (we dropped the index
“incident” and “incidence”):
Drag bat inertial viscous
The lift and drag coefficients and their ratio:
– In order to calculate and chart the polar coordinate we F
Lift
Lift 2
need to calculate the lift and drag coefficients which are
wing
essentially the lift and drag forces normalized to the area of
the wing, the density of the air and the square of the speed. F
Drag
Drag 2
Their definitions are (we replaced Abat with Awing) :
inertial viscous
The ratio of the two
Lift
coefficients is the
gliding ratio and it’s
inertial viscous
a useful formula. The
Drag
final lift and drag
coefficients and
Lift viscous
their ratio are:
Drag inertial viscous
Conclusions
– A ping-pong analogy was used to estimate the lift and
drag forces and the corresponding coefficients of a flat, thin
airfoil in a “ping-pong paddle” model. These formulas will
later be used to chart the polar diagrams of the same foil.
– Even though the assumptions involved are overly
simplified in nature and have seemingly not that much to
do with real aerodynamics, the basic physics of the paddle
ball interaction were modeled correctly and we will later
see that the resulting polar diagrams will look pretty
similar to the real ones for a thin flat foil.
– The simplified assumptions left no room for any estimate
of the angular momentum on a wing or modeling what
happen on the top of the wing (including stall).
– We will later use these formulas to model a virtual glider. Example polar coordinates for a performance hang glider
to be continued…